Get Started in Markdown

Here we will see how we can make a Mandelbrot set plot with python code.

Important

This exercise focuses on intermediate level python users who feel comfortable using numpy and complex numbers

MandelBrot set

The Mandelbrot set is a beautiful fractal and is defined as the set of complex numbers \(c\) for which the sequence \(z_{n+1} = z_n^2 + c\) does not diverge when iterated from \(z=0\), i.e., for which the sequence \(|z_n|\) remains bounded in value.

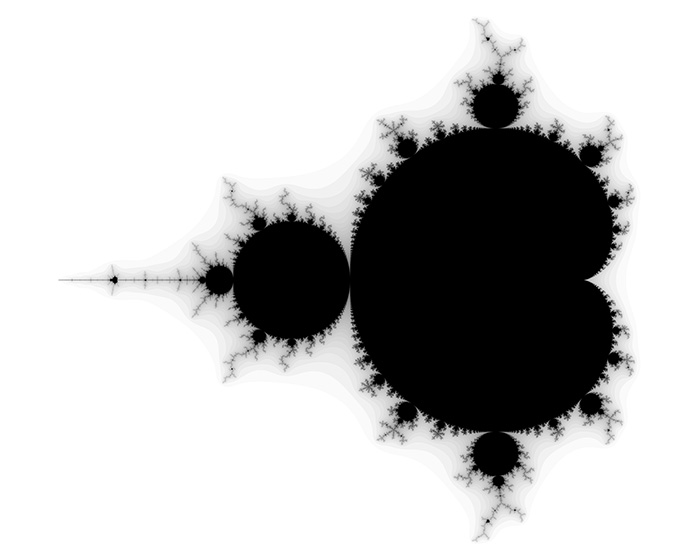
Full Code

import numpy as np
import matplotlib.pyplot as plt


def mandelbrot(c, max_iter) -> int:
    """
    Computes the number of iterations required for the complex number c to escape the Mandelbrot set.

    Args:
        c (complex): The complex number to test.
        max_iter (int): The maximum number of iterations to perform.

    Returns:
        int: The number of iterations required for c to escape the Mandelbrot set, or 0 if it does not escape within max_iter iterations.
    """
    z = 0
    n = 0
    while abs(z) <= 2 and n < max_iter:
        z = z*z + c
        n += 1
    if n == max_iter:
        return 0
    else:
        return n


def mandelbrot_set(xmin, xmax, ymin, ymax, width, height, max_iter) -> tuple:
    """
    Computes the Mandelbrot set for a given range of complex plane coordinates.

    Args:
        xmin (float): The minimum value of the x-axis.
        xmax (float): The maximum value of the x-axis.
        ymin (float): The minimum value of the y-axis.
        ymax (float): The maximum value of the y-axis.
        width (int): The number of pixels in the x-axis.
        height (int): The number of pixels in the y-axis.
        max_iter (int): The maximum number of iterations to compute the Mandelbrot set.

    Returns:
        tuple: A tuple containing the x-axis values, y-axis values, and the computed pixels.
    """
    x = np.linspace(xmin, xmax, width)
    y = np.linspace(ymin, ymax, height)
    pixels = np.zeros((height, width))
    for i in range(height):
        for j in range(width):
            pixels[i, j] = mandelbrot(x[j] + 1j*y[i], max_iter)
    return (x, y, pixels)


def plot_mandelbrot(x, y, pixels) -> None:
    """
    Plots the Mandelbrot set using the given pixel values.

    Args:
        x (numpy.ndarray): Array of x-coordinates.
        y (numpy.ndarray): Array of y-coordinates.
        pixels (numpy.ndarray): Array of pixel values.

    Returns:
        None
    """
    plt.imshow(pixels.T, cmap='hot', extent=(y.min(), y.max(), x.min(), x.max()))
    plt.xlabel('Real')
    plt.ylabel('Imaginary')
    plt.show()


# Example usage
def main():
    xmin, xmax = -2, 1
    ymin, ymax = -1.5, 1.5
    width, height = 1000, 1000
    max_iter = 100
    x, y, pixels = mandelbrot_set(xmin, xmax, ymin, ymax, width, height, max_iter)
    plot_mandelbrot(x, y, pixels)


if __name__ == '__main__':
    main()

Code Explanation

Hyperlink test

Please see mandelbrot_maker.mandelbrot()

Autofunction test

.. py:function:: mandelbrot(c, max_iter) -> int :no-index: :module: mandelbrot_maker

Computes the number of iterations required for the complex number c to escape the Mandelbrot set.

Args: c (complex): The complex number to test. max_iter (int): The maximum number of iterations to perform.

Returns: int: The number of iterations required for c to escape the Mandelbrot set, or 0 if it does not escape within max_iter iterations.